Question

10)_

If you multiply six positive numbers, the product's sign will be . If you mump
negative numbers, the product's sign will be
a) Positive; Negative
b) Negative; Negative
c) Positive; Positive
d) Negative; Positive
on will be. If you multiply six

Answer 1

c) Positive; Positive

Step-by-step explanation:

As we know it is a basic rule in mathematics, that if positive is multiplied by positive it gives a positive result always. So hence, from this rule it is proved that if we multiply 6 positive numbers, we will be getting the product which will be having positive sign. And in the second part of the questions, answer is that if we multiply 6 negative numbers then the sign remains positive as we know it is also a basic rule in mathematics that when 2 negative numbers are multiplied by each and other then it will give a positive product. So the answer will remain 'c) Positive; Positive''

Answer 2

Step-by-step explanation:

Step-by-step explanation:

The product of two positive numbers is always positive.

So product of All positive numbers will be always positive.

For Example

( + ) × ( + ) = ( + )

The product of two negative number is always positive.

So product of even negative numbers will be always positive

For example

( - ) × ( - ) = ( + )

Also product of negative and positive is also given as a negative

( - ) × ( + ) = ( - )

and

( + ) × ( - ) = ( - )

Let the Six Positive numbers be

a, b ,c , d, e, f

So the Product of Six Positive numbers will be

`(+a)* (+b)* (+c)* (+d)* (+e)* (+f)=\textrm{Positive Number}`

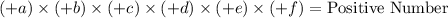
Let the Six Negative numbers be

-a, -b ,-c , -d, -e, -f

So the Product of Six Negative numbers will be

`(-a)* (-b)* (-c)* (-d)* (-e)* (-f)=\textrm{Positive Number}`

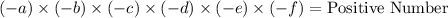
Therefore the Product's Sign will be

a) Positive ; Positive