Question

A rectangular poster is 3 times as long as it is wide. A rectangular banner is 5 times as long as it is wide. Both the banner and the poster have perimeters of 24 inches. What are the lengths and wides of the poster and the poster?

Answer 1

Length of poster is 9 inches and width of the poster is 3 inches.

Length of banner is 10 inches and width of the banner is 2 inches.

Explanation:

Given:

Perimeter of Banner =24 in.

Perimeter of poster = 24 in.

we need to find the dimensions of poster and banner.

First we will find the dimension of poster.

Now Given:

A rectangular poster is 3 times as long as it is wide.

Let the Width of poster be
`'p'`.

Length of the poster =
`3p`

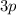
Perimeter of poster = 24 in.

But perimeter of poster is equal to twice the sum of length and width.

framing in equation form we get;

`2(p+3p)=24\\\\2(4p)=24\\\\8p=24\\\\p=(24)/(8) = 3\ in.`

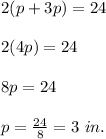
Now width of poster = 3 inches

Length of the poster =
`3p = 3*3 =9\ inches`

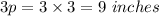
Hence Length of poster is 9 inches and width of the poster is 3 inches.

Now we will find the dimension of Banner.

Now Given:

A rectangular banner is 5 times as long as it is wide.

Let the Width of Banner be
`'b'`.

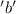Length of the banner =
`5b`

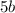
Perimeter of banner = 24 in.

But perimeter of banner is equal to twice the sum of length and width.

framing in equation form we get;

`2(b+5b)=24\\\\2(6b)=24\\\\12b=24\\\\b=(24)/(12) = 2\ in.`

Now width of banner = 2 inches

Length of the banner =
`5b = 5*2 = 10\ inches`

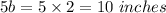
Hence Length of banner is 10 inches and width of the banner is 2 inches.