Answer : The vapor pressure of
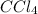 at
at
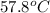 is 0.519 atm.
is 0.519 atm.
Explanation :
The Clausius- Clapeyron equation is :
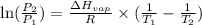
where,
 = vapor pressure of
= vapor pressure of
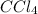 at
at
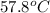 = ?
= ?
 = vapor pressure of
= vapor pressure of
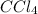 at normal boiling point = 1 atm
at normal boiling point = 1 atm
 = temperature of
= temperature of
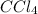 =
=
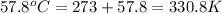
 = normal boiling point of
= normal boiling point of
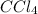 =
=
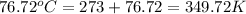
 = heat of vaporization
= heat of vaporization
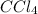 = 33.05 kJ/mole = 33050 J/mole
= 33.05 kJ/mole = 33050 J/mole
R = universal constant = 8.314 J/K.mole
Now put all the given values in the above formula, we get:
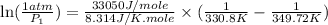
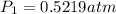
Hence, the vapor pressure of
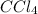 at
at
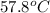 is 0.519 atm.
is 0.519 atm.