Answer:
(–3, –2)
(–1, –2)
(1, –2)
Explanation:
we know that
If a ordered pair is a solution of the linear inequality, then the ordered pair must satisfy the inequality. The ordered pair must lie on the shaded are of the solution set
we have
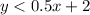
The solution of the inequality is the shaded area below the dashed line

Verify each ordered pair
Substitute the value of x and the value of y in the inequality and then compare the results
case 1) (–3, –2)
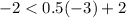
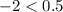 ----> is true
----> is true
so
The ordered pair satisfy the inequality
therefore
The ordered pair is a solution of the inequality (the ordered pair lie on the shaded area of the solution set)
case 2) (–2, 1)
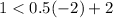
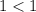 ----> is not true
----> is not true
so
The ordered pair not satisfy the inequality
therefore
The ordered pair is not a solution of the inequality (the ordered pair not lie on the shaded area of the solution set)
case 3) (–1, –2)
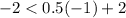
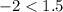 ----> is true
----> is true
so
The ordered pair satisfy the inequality
therefore
The ordered pair is a solution of the inequality (the ordered pair lie on the shaded area of the solution set)
case 4) (–1, 2)
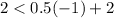
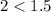 ----> is not true
----> is not true
so
The ordered pair not satisfy the inequality
therefore
The ordered pair is not a solution of the inequality (the ordered pair not lie on the shaded area of the solution set)
case 5) (1, –2)
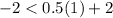
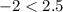 ----> is true
----> is true
so
The ordered pair satisfy the inequality
therefore
The ordered pair is a solution of the inequality (the ordered pair lie on the shaded area of the solution set)
see the attached figure to better understand the problem