Question

Help please!

A cat is chasing a mouse across a 1.3 m tall dining table. The mouse darts to the side and the cat accidentally slides off, landing clumsily 0.75 m away from the edge of the table. How long did it take the cat to hit the ground?
0.78 seconds
0.51 seconds
0.27 seconds
0.95 seconds

Using the information you solved for in problem 9, how fast was the cat moving when it fell off the table?
2.97 m/s
1.45 m/s
3.81 m/s
6.93 m/s

Answer 1

The will hit the ground in t = 0.51[sg] and he was moving with a velocity of 1.45 [m/s]

Step-by-step explanation:

In the attached image, we can see a sketch of the conditions of the problem, we are interested to know how long the cat takes to fall from the table to the ground and then using that time we can find the initial velocity of the cat when it arrives at the edge of the table.

The initial condition of this problem is that when the cat reaches the edge of the table, it will only have initial velocity on the X-axis component, its velocity on the y-axis component will be zero.

Therefore:

`y=(v_(y))_(0)`

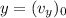
In this way, we can use the following equation of kinematics and find the time t.

`y=(v_(y))_(0)*t - (1)/(2) * g*t\\where:\\g =9.81[m/s^(2) ] gravity\\y = 1.3 [m] table height` =0[/tex]

We note that gravity is taken as a negative quantity since the movement is downwards, likewise the value and the equation will have negative value as the reference point will be taken as the edge of the table, therefore, y = -1.3 [m]

`-1.3 = (0)*t - (1)/(2) * (9.81)*t^(2) \\t = \sqrt{(2*1.3)/(9.81) }\\ t= 0.51 [m/s]\\`

Now using this time and replacing in the next kinematics equation we will have:

`x=(v_(x) )_(0) *t\\where\\x=0.75 [m]`

We will have:

`x=(v_(x) )_(0) *t\\\\(v_(x) )_(0)=(x)/(t) \\(v_(x) )_(0)=(0.75)/(0.51)\\ (v_(x) )_(0)=1.45 [m/s]`