Question

Find all roots x^3 + 7x^2 + 12x = 0

Answer 1

Therefore the THREE roots are

`x=0\ or\ x=-3\ or x=-4`

Explanation:

Given:

`x^(3)+7x^(2) +12x= 0`

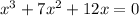
To Find:

All the Roots = ?

Solution:

As the degree of the polynomial is THREE then the number of root are also THREE.

`x^(3)+7x^(2) +12x= 0\\\\x(x^(2)+7x +12)= 0\\\\x=0\\or\\x^(2)+7x +12= 0\\`

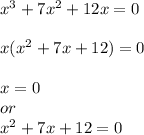
Now one root is Zero For other we need to Factorize

So by Splitting the middle term

i.e Factor of 12 such that sum should be 7

i.e 3 × 4 = 12 and 3 + 4 = 7

∴
`x^(2)+7x +12= 0\\x^(2)+3x+4x +12= 0\\x(x+3)+4(x+3)=0\\(x+3)(x+4)=0\\\\x+3=0\ or\ x+4 = 0\\\\\therefore x=-3\ or x=-4\ \textrm{Which are the roots}`

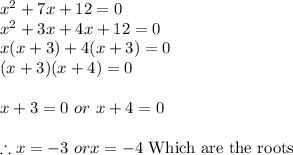
Therefore the THREE roots are

`x=0\ or\ x=-3\ or x=-4`