Answer:
6. Acceleration = 4.74 m/s^2
7. Centripetal force = 40.5 N
Step-by-step explanation:
Problem 6.
Recall that the centripetal acceleration is defined as:
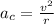 , where V is the object's tangential velocity, and r the radius of the circular motion. Therefore, in or case, the centripetal acceleration would be:
, where V is the object's tangential velocity, and r the radius of the circular motion. Therefore, in or case, the centripetal acceleration would be:
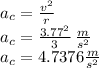
which we can round to 4.74 m/s^2 (option b in your list)
Problem 7.
Now we need to find not just the centripetal acceleration using the same formula as above, but then the centripetal force.
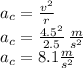
Now we calculate the centripetal force by multiplying this acceleration times the mass of the object following the definition of force as mass times acceleration:
Centripetal force = 5.0 kg * 8.1 m/s^2 = 40.5 N
The answers comes in Newtons (N)