Question

If f (x) = 3 x squared and g (x) = 4 x cubed + 1, what is the degree of (f circle g) (x)?If f (x) = 3 x squared and g (x) = 4 x cubed + 1, what is the degree of (f circle g) (x)?

options are
2
3
5
6

Answer 1

the answer is 6

Explanation:

don't have one

Answer 2

degree = 6

Explanation:

Given
`f(x)=3x^2`, and
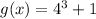`g(x)=4^3+1`, we can find the composition of functions:
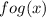`fog(x)` by applying the definition of composition and performing the needed algebra.

Recall that the composition of functions is defined as:
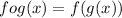`fog(x)=f(g(x))`, where we use as input for the function f(x) the actual expression in terms of "x" of the function g(x):

`f(g(x))=f(4x^3+1)\\f(g(x))=3(4x^3+1)^2\\f(g(x))=3\,(4x^3+1)\,(4x^3+1)\\f(g(x))=3\,[16x^6+4x^3+4x^3+1]\\f(g(x))=3\,[16x^6+8x^3+1]\\f(g(x))=48x^6+24x^3+3`

Therefore, the degree of this expression is "6" (the highest power at which the variable "x" appears)