Answer:
John work as a security guard job 27 hours and as a landscaper 33 hours.
Explanation:
Given:
John works two jobs.
As a security guard he earns $8.50 per hour and as a landscaper he earns $14 per hour.
One week John worked a total of 60 hours and earned $691.50.
Now, to find hours he work at each job.
Let the job of security guard be
 hours.
hours.
And the job of landscaper be
 hours.
hours.
So, the total hours John worked in a week:
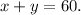
⇒
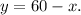 ............( 1 )
............( 1 )
Now, the money earned by John in a week:
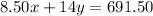
Putting the equation ( 1 ) in the place of
 :
:
⇒

⇒
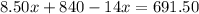
Moving variables on one side and the other we get:
⇒

⇒
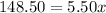
Dividing both sides by 5.50 we get:
⇒
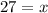
As a security guard job he worked 27 hours.
Putting the value of in
 equation ( 1 ) we get:
equation ( 1 ) we get:
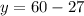
⇒

As a landscaper he worked 33 hours.
Therefore, John work as a security guard job 27 hours and as a landscaper 33 hours.