Question

When Julia is writing a first draft, there is 0.7 probability that there will be no spelling mistakes on a page. One day, Julia writes a first draft that is 4 pages long. Assuming that Julia is equally likely to have a spelling mistake on each of the 4 pages, what is the probability that she will have no spelling mistakes on at least one of them?

Answer 1

real answer is 0.9919 but it depends if needed to round it is .99

Explanation:

it was khan academy algebra 2 assignement

Answer 2

The required probability is given by, 0.9919.

Explanation:

Let, X be the random variable denoting the no. of pages among those 4 pages which Julia writes where she makes no spelling mistake.

clearly,

X
`\sim` Binomial (4, 0.7)

So, P(X = x) =
`^4C_(x) * (0.7)^(x) * (0.3)^((4 - x))`

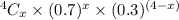
[when x = 0, 1, 2, 3, 4]

= 0 otherwise

According to the question, we are to find out P(X ≥ 1) .

Now, P(X ≥ 1)

= 1 - P(X = 0)

=
`1 - (^4C_(0) * (0.7)^(0) * (0.3)^(4))`

=
`1 - 0.0081`

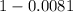
= 0.9919

So, the required probability is given by, 0.9919