Question

Simplify the expression state any excluded values
2a^2-4a+2
---------------
3a^2-3

Answer 1

3

Explanation:

Answer 2

The simplified form is
`(2(x-1))/(3(x+1))`.

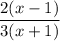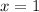`x =1` is the excluded value for the given expression.

Explanation:

Given:

The expression given is:

`(2a^2-4a+2)/(3a^2-3)`

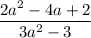
Let us simplify the numerator and denominator separately.

The numerator is given as
`2a^2-4a+2`

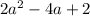
2 is a common factor in all the three terms. So, we factor it out. This gives,

`=2(a^2-2a+1)`

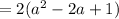
Now,
`a^2-2a+1=(a-1)(a-1)`

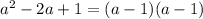
Therefore, the numerator becomes
`2(a-1)(a-1)`

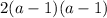
The denominator is given as:
`3a^2-3`

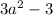
Factoring out 3, we get

`3(a^2-1)`

Now,
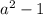`a^2-1` is of the form
`a^2-b^2=(a-b)(a+b)`

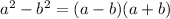
So,
`a^2-1=(a-1)(a+1)`

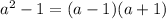
Therefore, the denominator becomes
`3(a-1)(a+1)`

Now, the given expression is simplified to:

`(2a^2-4a+2)/(3a^2-3)=(2(x-1)(x-1))/(3(x-1)(x+1))`

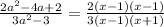
There is
`(x-1)` in the numerator and denominator. We can cancel them only if
`x\\e1` as for
`x=1`, the given expression is undefined.

Now, cancelling the like terms considering
`x\\e1`, we get:

`(2a^2-4a+2)/(3a^2-3)=(2(x-1))/(3(x+1))`

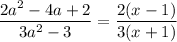
Therefore, the simplified form is
`(2(x-1))/(3(x+1))`

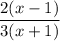
The simplification is true only if
`x\\e1`. So,
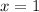`x =1` is the excluded value for the given expression.