Question

A clinical trial tests a method designed to increase the probability of conceiving a girl. In the study 340 babies were​born, and 306 of them were girls. Use the sample data to construct a 99​% confidence interval estimate of the percentage of girls born. Based on the​ result, does the method appear to be​ effective?

? < P < ?

Does the method appear to be​ effective?

​A. No, the proportion of girls is not significantly different from 0.5.
B. Yes, the proportion of girls is significantly different from 0.5

Answer 1

B. Yes, the proportion of girls is significantly different from 0.5

Explanation:

Given that a clinical trial tests a method designed to increase the probability of conceiving a girl. In the study 340 babies were​born, and 306 of them were girls.

Sample proportion =
`(306)/(340) \\=0.9`

Std error of p =
`\sqrt{(pq)/(n) } \\=0.01627`

For sample size large, we find that t distribution almost coincides with z distribution

Hence 99% critical value = 2.58

Margin of error = 2.58*std error = 0.0420

Confidence interval =
`(0.9-0.0420, 0.9+0.0420)`

=
`(0.858, 0.942)`

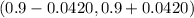
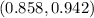
0.858<p<0.942

Yes, this method is effective becuse lower bound itself is 0.858 i.e. there is 85.8%chance for getting girls in birth

B. Yes, the proportion of girls is significantly different from 0.5