Answer:
y = 9.64 m
Step-by-step explanation:
This exercise should be solved using kinematics in one dimension, let's write the equations for the two cases presented
The rock is released
y = y₀ + V₀₁ t₁ - ½ g t₁²
In this case the speed starts is zero
y = y₀ - ½ g t₁²
The rock is thrown up
y = y₀ + v₀² t₂ -½ g t₂²
The height that reaches the floor is zero
y₀ - ½ g t₁² = y₀ + v₀₂ t₂ - ½ g t₂²
We use the initial velocity with the equation
v₂² = v₀₂² - 2 g y
At the point of maximum height v₂ = 0
v₀₂ = √ (2 g
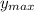 )
)
g (-t₁² + t₂²) = 2 √ (2 g
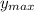 ) t₂²
) t₂²
g (- 4.15² + 6.30²) = 2 √ (2 2 g) 6.3
g (22.4675) = 25.2 √ g
g² = 2²5.2 / 22.4675 g
g = 1.12 m / s²
Having the value of g we can use any equation to find the height
y = ½ g t₁²
y = ½ 1.12 4.15²
y = 9.64 m