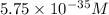Answer: The ratio of
![[Cu^(2+)]/[Cu^+]](https://img.qammunity.org/2020/formulas/chemistry/middle-school/17i2b90tikw4r9z1c9qd38k9quip9egqyu.png) in an aqueous solution is
in an aqueous solution is
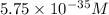
Step-by-step explanation:
The substance having highest positive
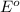 potential will always get reduced and will undergo reduction react.
potential will always get reduced and will undergo reduction react.
Oxidation half reaction:
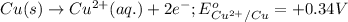
Reduction half reaction:
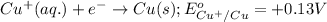 ( × 2)
( × 2)
Net cell reaction:
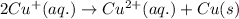
Substance getting oxidized always act as anode and the one getting reduced always act as cathode.
To calculate the
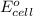 of the reaction, we use the equation:
of the reaction, we use the equation:
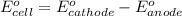
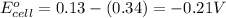
To calculate the EMF of the cell, we use the Nernst equation, which is:
![E_(cell)=E^o_(cell)-(0.059)/(n)\log ([Cu^(2+)])/([Cu^(+)]^2)](https://img.qammunity.org/2020/formulas/chemistry/middle-school/iddt2zum075yqi3yrzhel0con8dqb6v35s.png)
Concentration of pure solids and liquids are taken as 1 in the ratio
where,
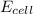 = electrode potential of the cell = +0.80 V
= electrode potential of the cell = +0.80 V
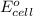 = standard electrode potential of the cell = -0.21 V
= standard electrode potential of the cell = -0.21 V
n = number of electrons exchanged = 2
![([Cu^(2+)])/([Cu^+]^2)](https://img.qammunity.org/2020/formulas/chemistry/middle-school/3ldx9z1tons1trfbn8mgrmvqnwisb3p1bm.png) = Ratio of the concentration
= Ratio of the concentration
Putting values in above equation, we get:
![0.80=-0.21-(0.059)/(2)* \log(([Cu^(2+)])/([Cu^(+)]^2))\\\\([Cu^(2+)])/([Cu^(+)]^2)=5.75* 10^(-35)M](https://img.qammunity.org/2020/formulas/chemistry/middle-school/52vxhyp91xi799h5x08l4omjyrgdpyks1z.png)
Hence, the ratio of
![[Cu^(2+)]/[Cu^+]](https://img.qammunity.org/2020/formulas/chemistry/middle-school/17i2b90tikw4r9z1c9qd38k9quip9egqyu.png) in an aqueous solution is
in an aqueous solution is