Answer:
DNE
Explanation:
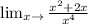
As you can see in the picture I attached to this, that as the limit goes to zero from the negative side, it approaches -∞ and from the positive side, it approaches ∞ . hence, the limit doesn't exist.
To show this algebraically, you have to imagine how a number divided by zero looks like, Just know the graph of 1/x and see how the limit to zero doesnot exist. I hope this helps!