Question

A recent survey of 50 executives who were laid off during a recent recession revealed it took a mean of 26 weeks for them to find another position. The standard deviation of the sample was 6.2 weeks. Construct a 95% confidence interval for the population mean. Is it reasonable that the population mean is 28 weeks? Justify your answer.

Answer 1

Answer with explanation:

Formula to find the confidence interval for population mean :-

`\overline{x}\pm t^*(\sigma)/(√(n))`

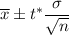
, where
`\overline{x}` = sample mean.

t*= critical z-value

n= sample size.

s= sample standard deviation.

By considering the given question , we have

`\overline{x}= 26`

`s=6.2`

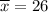
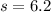
n= 50

Degree of freedom : df = 49 [ df=n-1]

Significance level :
`\alpha=1-0.95=0.05`

Using students's t-distribution table, the critical t-value for 95% confidence =
`t_(\alpha/2,df)=t_(0.025,49)=2.010`

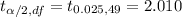
Then, 95% confidence interval for the population mean will be :

`26\pm (2.010)(6.2)/(√(50))`

`=26\pm (2.010)(6.2)/(7.0710)`

`=26\pm (2.010)(0.87682)`

`\approx26\pm1.76`

`=(26-1.76,\ 26+1.76)=(24.24,\ 27.76)`

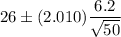
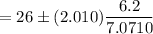
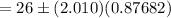
Hence, a 95% confidence interval for the population mean = (24.24, 27.76)

Since 28 is not contained in the above confidence interval , it means it is not reasonable that the population mean is 28 weeks.