Answer:
The number of dimes is 22 and the number of nickels is 8.
Explanation:
Given:
Jaimes has $2.60 in dimes and nickels.
The number of dimes is 14 more than the number of nickels.
Now, to find the each coin he have.
Let the dimes be
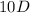 .
.
And the nickel be
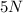 .
.
(As 1 dime = 10 cent and 1 nickel = 5 cent)
As given in question:
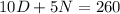 ............(1)
............(1)
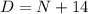 ...................(2) (The number of dimes is 14 more than number of nickels.)
...................(2) (The number of dimes is 14 more than number of nickels.)
Now, solving the equation (1):
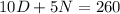
Putting the value of equation (2) on place D we get:
 .
.
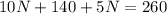 .
.
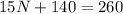 .
.
Subtracting both sides by 140 we get:
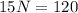 .
.
Dividing both sides by 15 we get:
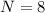 .
.
The number of nickels = 8.
And the number of dimes = (N+14) = 8+14=22.
Therefore, the number of dimes is 22 and the number of nickels is 8.