Question

A​ one-way road passes under an overpass in the shape of half an​ ellipse, 20 ft high at the center and 20 ft wide. Assuming a truck is 16 ft​ wide, what is the tallest truck that can pass under the​ overpass?\

Answer 1

To find the tallest truck that can pass under the overpass, the width of the truck is used to determine the maximum height available under an elliptical overpass. Using the equation of an ellipse, we can calculate the height clearance for a truck that is 16 ft wide.

Step-by-step explanation:

To determine the tallest truck that can pass under the overpass, we need to consider the dimensions of the half-ellipse representing the overpass and the width of the truck. The semi-major axis a is half the width of the overpass, and the semi-minor axis b is the height of the overpass at the center. The equation of the ellipse in standard form is (x^2 / a^2) + (y^2 / b^2) = 1. Here a = 10 ft and b = 20 ft. Given that the truck is 16 ft wide, it will pass through a point where x = 8 ft (half its width) from the center of the road.

To find the height at this point, we plug in the value x = 8 ft into the ellipse's equation and solve for y: (8^2 / 10^2) + (y^2 / 20^2) = 1. Solving for y, we find the maximum height at which the truck can pass under without hitting the overpass. Subtracting the height of the truck from the height of the overpass will give us the tallest truck that can safely make it through without collision.

Answer 2

The "tallest truck" that it passes by is 12 ft.

Step-by-step explanation:

Given that,

The truck passes through "Height of an half ellipse" is 20 ft ("a" is "Major axis").

The truck passes through "Width of an ellipse" is 20 ft ("b" is "Minor axis").

The width of the truck is 16 ft (x).

We need to find the greatest "height of the truck" (y) which enters into an ellipse.

We know that,

Equation of ellipse is
`\frac {x^2}{b^2}+ \frac {y^2}{a^2}=1`

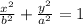
Substitute the given values in the above formula,

`\frac {16^2}{20^2}+ \frac {y^2}{20^2}=1`

`\frac {256}{400}+ \frac {y^2}{400}=1`

`0.64+ \frac {y^2}{400}=1`

`\frac {y^2}{400}=1-0.64`

`\frac {y^2}{400}=0.36`

`y^2=0.36*400`

`y^2=144`

`y=√(144)`

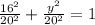
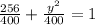
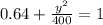
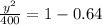
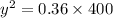
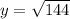
y = 12

Therefore, The "tallest truck" that it passes by is 12 ft.