Answer:
ONE SOLUTION
Explanation:
When two points on a line are given, the equation of the line is given by the formula:
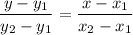
where
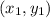 and
and
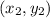 are the points on the line.
are the points on the line.
Here, the first set of points are:
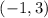 and
and
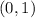 .
.
Therefore,
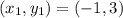 and
and
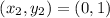 .
.
The line passing through this is given by:
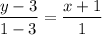
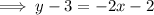
∴ 2x + y - 1 =0
Now, for the second line, the points are:
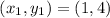 and
and
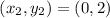 .
.
Therefore,

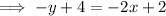
∴ 2x - y + 2 = 0
Now, to determine the number of solutions the two equations have, we solve these two equations,
Adding Eqn(1) and Eqn(2) we get:
4x = -1
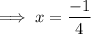
And
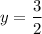 .
.
Since, we arrive at unique values of 'x' and 'y', we say the lines have only one unique solution.