Question

Two sides of a triangle are 8 m and 9 m in length and the angle between them is increasing at a rate of 0.06 rad/s. Find the rate at which the area of the triangle is increasing when the angle between the sides of fixed length isπ3rad.

Answer 1

Rate at which area is increasing is 1.08 m²/s.

Explanation:

Area of triangle is with side a and b and angle C between them is given by

A = 0.5 ab SinC

Here we need to find how area changes with a and b fixed and C is changing,

`(dA)/(dt)=(d)/(dt)\left (0.5 absinC\right )\\\\(dA)/(dt)=0.5ab(d)/(dt)\left (sinC\right )\\\\(dA)/(dt)=0.5abcosC(dC)/(dt)`

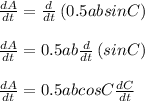
We have

a = 8 m

b = 9 m

`C=(\pi)/(3)rad\\\\(dC)/(dt)=0.06rad/s`

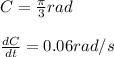
Substituting

`(dA)/(dt)=0.5* 8* 9* cos\left ( (\pi)/(3)\right )* 0.06\\\\(dA)/(dt)=1.08m^2/s`

Rate at which area is increasing is 1.08 m²/s.