Question

From a Group of 8 People, Including George and Nina, 3 people are to be selected at random to work on a certain project. What is the probability that 3 people selected will include George but not NinaA 5/56B 9/56C 15/56D 21/56E 25/56

Answer 1

Answer: C.
`(15)/(56)`

Step-by-step explanation:

Given : Total people = 8

Number of people are to be selected = 3

The number of combinations of r things taken out of n things is given by :-

`^nC_r=(n!)/(r!(n-r)!)`

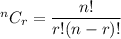
The total number of ways to select 3 people out of 8 is given by :-

`^8C_3=(8!)/(3!(8-3)!)\\\\=(8*7*6*5!)/(3!*5!)=(8*7*6)/(3*2*1)=56`

If George is included , then one person is confirmed, so we need to selec only 2 people out of 7.

Also, Nina is not selected , so the total number of people left= 6

The total number of ways to select 2 people out of 6 that will include George but not Nina is given by :-

`^6C_2=(6!)/(2!(6-2)!)\\\\=(6*5*4!)/(2!*4!)=(30)/(2)=15`

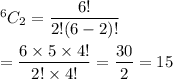
i.e. No. of favorable outcomes= 15

Now, the probability that 3 people selected will include George but not Nina :-

`\frac{\text{Favorable outcomes}}{\text{Total outcomes}}\\\\=(15)/(56)`

Hence, the required probability = C.
`(15)/(56)`