Question

How much would $600 be worth after 10 years, if it were invested at 4%

interest compounded continuously? (Use the formula below and round your
answer to the nearest cent.)
Aft) = Poet

Answer 1

The worth after 10 years if it were invested at 4% interest compounded continuously is $ 895.094

Solution:

Given that $ 600 invested at 4 % interest compounded continously for 10 years

To find: total amount after 10 years

The compound interest formula for compounded continously is given as:

`A = p e^(rt)`

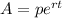
Where "p" is the principal

"r" is the rate of interest

"t" is the number of years

Here in this problem, p = 600

`r = 4 \% = (4)/(100) = 0.04`

t = 10 years

Substituting the values in formula we get,

`A = 600 e^(0.04 * 10)\\\\A = 600 e^(0.4)\\\\A = 600 * 1.49182469764\\\\A = 895.094`

Thus the worth after 10 years is $ 895.094