Question

Suppose a simple random sample of size n = 1000 is obtained from a population whose size is N = 2,000,000 and whose population proportion with a specified characteristic is p = 0.49 . What is the probability of obtaining x = 520 or more individuals with the​ characteristic?

Answer 1

P(X≥520) =0.02938

Explanation:

given,

n = 1000

Population size = N = 2,000,000

Specified characteristic = P = 0.49

Probability of obtaining x = 520

`\sigma_p = \sqrt{(p(1-p))/(1000)}`

`\sigma_p = \sqrt{(0.49(1-0.49))/(1000)}`

`\sigma_p =0.0158`

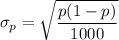
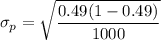
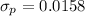
for x = 520

p = 520/1000 = 0.52

P(X≥520) = P(p≥0.52)

P(p≥0.52) =
`P(Z\geq (0.52-0.49)/(0.0158))`

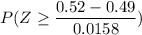
P(p≥0.52) =
`P(Z\geq 1.898)`

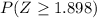
using z-table

P(p≥0.52) = 0.02938

P(X≥520) =0.02938

Answer 2

`p(x\geq 520)  = 0.0293`

Explanation:

Given data:

random sample size n = 1000

Population size is N - 2,000,000

P = 0.49

We know

`\sigma_p = \sqrt{(p*(1-p))/(n)}`

`\sigma_ p = \sqrt{(0.49*(1-0.49))/(1000)} = 0.0158`

Probability for having X =520

sample proportion
`\hat p = (520)/(1000) = 0.52`

`p(x\geq 520)  = P(\hat p\geq)`

`= P(Z\geq (0.52 - 0.49)/(0.0158))`

`= P(Z\geq 1.89) = 0.0293`

`p(x\geq 520)  = 0.0293`