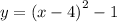Question

The vertex is (4, -1) and contains the point (2, 3)

Answer 1

`y = {(x - 4)}^(2) - 1`

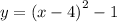
Explanation:

`y = a(x - h)^(2) + k`

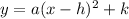
We know the value of h and k.

`y = a(x - 4)^(2) - 1`

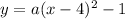
The graph passes through (2,3). Therefore, substitute x = 2 and y = 3.

`3 = a {(2 - 4)}^(2) - 1`

Solve for a.

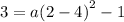
`3 = a {( - 2)}^(2) - 1 \\ 3 = 4a - 1 \\ 3 + 1 = 4a \\ 4 = 4a \\ (4)/(4) = a \\ 1 = a`

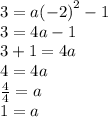
Therefore, a = 1. Rewrite the equation.

`y = 1 {( x - 4)}^(2) - 1 \\ y = {(x - 4)}^(2) -1`

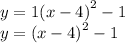
Answer Check

Substitute x = 2 and y = 3 in the equation.

`3 = {(2 - 4)}^(2) - 1 \\ 3 = {( - 2)}^(2) - 1 \\ 3 = 4 - 1 \\ 3 = 3`

The equation is true for (2,3). Therefore, the answer is —

`y = {(x - 4)}^(2) - 1`