Question

URGENT!!!

Find the equation x^2 + y^2 + Dx + Ey + F = 0
of the circle that passes through the points. To verify your result, use a graphing utility to plot the points and graph the circle.
(0, 0), (8, 8), (16, 0)

Answer 1

D= -16

E= 0

F= 0

Explanation:

The given equation is
`x^(2) + y^(2) + Dx + Ey + F = 0`

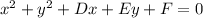
It is also given that the circle passes through (0,0) (16,0) and (8,8).

Inserting (0,0) in the equation, it gives

`0 + 0 + 0 + 0 + F = 0`

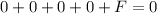
This gives F = 0 .

Now inserting (16,0) , it gives

`16^(2) + 0^(2) + D(16) + E(0) + 0 = 0`

`D(16) = -256`

`D = (-256)/(16)`

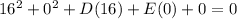
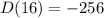
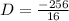
D = -16

Now inserting (8,8) , it gives

`8^(2) + 8^(2) + (-16)(8) + (E)(8) + 0 = 0`

`-16 + E = -16`

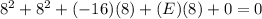
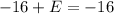
E = 0

Thus the equation of circle is

`x^(2) + y^(2) + (-16)x  = 0`

We can draw the following graph and thus verify that points (0,0) (8,8) and (16,0) lie on graph.