Given:
Two rational numbers are
 and
and
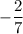 .
.
To find:
The three rational numbers between the given two numbers.
Solution:
Two rational numbers are
 and
and
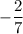 . First we need to make denominators common.
. First we need to make denominators common.
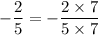

and,
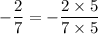
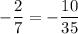
Now, we have same denominators. Numerators are -14 and -10.
So, three numbers between -14 and -10 are -11, -12 ,13.


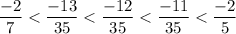
Therefore, the three rational numbers between
 and
and
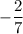 are
are
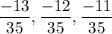 .
.