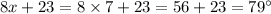Question

Urgent!!! Triangle ABC has angles the following angle measures. m<A=2x+7 , m<B=10x+10 and m<C=8x+23. what is the value of x and what is the measure of each angle?

x=
m<A=
m<B=
m<C=​

Answer 1

The Value of x= 7.

m∠A= 21°

m∠B= 80°

m∠C= 79°

Explanation:

Given:

m∠A = 2x+7

m∠B = 10x+10

m∠C = 8x+23

We need to find the value of x and measures of each angle.

Now by angle sum property which states that sum of all angles of a triangle are 180°.

Hence from above statement we can say in ΔABC;

m∠A+m∠B+m∠C=180

Now substituting the given values in above equation we get;

`2x+7+10x+10+8x+23=180\\2x+10x+8x+7+10+23=180\\20x+40=180\\20x=180-40\\20x=140\\\\x=(140)/(20) =7`

Hence value of x is 7.

Substituting the value of x in all angles we get;

m∠A=
`2x+7=2*7+7=14+7=21\°`

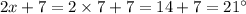
m∠B=
`10x+10=10*7+10=70+10=80\°`

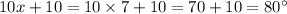
m∠C=
`8x+23= 8*7 +23 = 56+23=79\°`