Answer:
Perimeter of the smaller triangle = 1.75x
Explanation:
Let the two equal sides of an isosceles triangle = x
Therefore, length of the base =
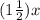
Perimeter of this isosceles triangle = x + x +
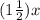
= 2x +
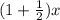
= 2x + x +
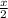
=
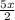
Since, smaller triangle has a perimeter that is half the perimeter of the larger triangle,
Length of each corresponding side of smaller triangle will be half of the larger triangle.
Length of sides of the smaller triangle =
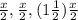
Perimeter of the smaller triangle =

= x +
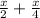
=
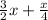
=
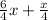
=
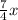
= 1.75x
Therefore, expression that represents the perimeter of the smaller triangle is (1.75x)