Question

A student dance committee is to be formed consisting of 2 boys and 4 girls. If the membership is to be chosen from 5 boys and 6 girls, how many different committees are possible?

Answer 1

150 different committees are possible

Solution:

Given that a student dance committee is to be formed consisting of 2 boys and 4 girls

The membership is to be chosen from 5 boys and 6 girls

To find : number of different possible committees

A combination is a selection of all or part of a set of objects, without regard to the order in which objects are selected

The formula for combination is given as:

`n C_(r)=(n !)/((n-r) ! r !)`

where "n" represents the total number of items, and "r" represents the number of items being chosen at a time

We have to select 2 boys from 5 boys

So here n = 5 and r = 2

`\begin{aligned} 5 C_(2) &=(5 !)/((5-2) ! 2 !)=(5 !)/(3 ! 2 !) \\\\ 5 C_(2) &=(5 * 4 * 3 * 2 * 1)/(3 * 2 * 1 * 2 * 1) \\\\ 5 C_(2) &=10 \end{aligned}`

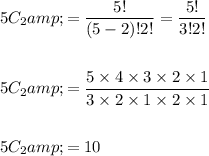
We have to select 4 girls from 6 girls

Here n = 6 and r = 4

`\begin{aligned} 6 C_(4) &=(6 !)/((6-4) ! 4 !)=(6 !)/(2 ! 4 !) \\\\ 6 C_(4) &=(6 * 5 * 4 * 3 * 2 * 1)/(2 * 1 * 4 * 3 * 2 * 1)=15 \end{aligned}`

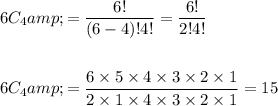
Committee is to be formed consisting of 2 boys and 4 girls:

So we have to multiply
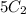`5 C_(2)` and
`6 C_(4)`

`5 C_(2) * 6 C_(4)=10 * 15=150`

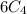
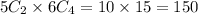
So 150 different committees are possible