Question

Suppose a dolphin sends out a series of clicks that are reflected back from the bottom of the ocean 65 m below. How much time elapses before the dolphin hears the echoes of the clicks? (The speed of sound in seawater is approximately 1530 m/s.)

Answer 1

To solve this problem it is necessary to apply the concepts related to the kinematic equations of motion.

By definition we know that speed can be expressed as

`v= (d)/(t)`

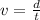
Where,

t = time

d = distance

For this specific case we know that the speed traveled corresponds to the round trip, therefore

`v = (2d)/(t)`

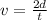
Re-arrange to find t (The time taken to here the echo)

`t = (2d)/(v)`

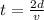
Replacing with our values we have that the distance is equal to 65m

`t = (2*65)/(1530)`

`t = 0.085s`

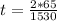
Therefore the time before the dolphin hears the echoes of the clicks is 0.085s