Question

The two representative points for the first half of a data in a data set are (1,10) and (8,2). Create a data set with at least eight points that fits these representative points and find the line of best fit for the data.

Answer 1

line : 8x+7y=78.

points: (0 , 78/7) ,

(78/8 , 0) ,

(2 , 62/7) ,etc.

Explanation:

• we have two points. so, we can find the equation of line passing through these 2 points

• the equation of a line passing through the two points P(a,b) and Q(c,d)

is :
`y-b=((d-b)/(c-a) )*(x-a)\\`

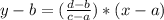
• here, the equation of line passing through the given two points is

`y-10=((2-10)/(8-1) )*(x-1)\\[tex]y-10=((-8)/(7) )*(x-1)`

• multiplying both sides by 7,

7y-70=-8x+8\\8x+7y=78[/tex]

• therefore, the line of best fit for the data is 8x+7y=78.

• now make 8 points which satisfy above line equation

( for easier way fix some value for x and substitute it in the above line equation then find corresponding y value. these x & y values will make a point. for more points , keep changing the values of x and find corresponding y values)

• (0 , 78/7) ,

(78/8 , 0) ,

(2 , 62/7) ,etc.