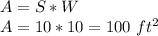Question

A farmer wants to fence a rectangular garden next to his house which forms the northern boundary. The fencingfor the southern boundary costs $6 per foot, and the fencing for the east and west sides costs $3 per foot. If hehas a budget of $120 for the project, what are the dimensions of the largest area the fence can enclose?

Answer 1

10 ft x 10 ft

Area = 100 ft^2

Explanation:

Let 'S' be the length of the southern boundary fence and 'W' the length of the eastern and western sides of the fence.

The total area is given by:

`A=S*W`

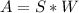
The cost function is given by:

`\$ 120 = \$3*2W+\$6*S\\20 = W+S\\W = 20-S`

Replacing that relationship into the Area function and finding its derivate, we can find the value of 'S' for which the area is maximized when the derivate equals zero:

`A=S*(20-S)\\A=20S-S^2\\(dA)/(dS) = (d(20S-S^2))/(dS)\\0= 20-2S\\S=10`

If S=10 then W =20 -10 = 10

Therefore, the largest area enclosed by the fence is:

`A=S*W\\A=10*10 = 100\ ft^2`