Answer: B.) Open circle on 2 and all the numbers to the right are shaded
Explanation:
The given inequality :

Divide both sides by 3 , we get
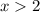
i.e. x is a numerical value strictly greater than 2 i.e. 2 is not-included.
When we plot this inequality on Number line , we starts with an open circle on 2 that signifies that 2 in not included and shade on the right side because x is greater than 2 which lies on the right side of 2 .
Hence, the correct answer is B.) Open circle on 2 and all the numbers to the right are shaded .