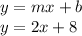Question

Line has the equation y=2x+2. Write the equation of the image of after a dilation with a scale factor of 4, centered at the origin.

Write your answer in slope-intercept form.

Answer 1

`y=2x+8`

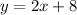
Explanation:

Given:

The original equation of the line 'l' is given as:

`y=2x+2`

Scale factor of dilation is,
`k=4`

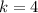
Now, when a line is dilated by some scale factor 'k' centered at the origin, then the parallelism of the line is reserved and only the y intercept changes.

The rule of dilation for a point
`(x,y)` with a scale factor 'k' is given as:

`(x,y)\rightarrow (kx,ky)`

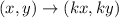
The standard form of a line is of the form
`y=mx+b`, where, 'm' is the slope and 'b' is the y-intercept of the line. On comparing the line 'l' with the standard form, we get:

`Slope,m=2\\y-intercept,b=2`

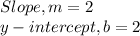
The y-intercept is at the point (0, 2). Now, applying dilation rules on this point, we get:

`(0,2)\rightarrow (4* 0,4* 2)=(0,8)`

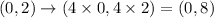
So, the new y-intercept after dilation is at (0, 8) or the new value of 'b' is 8.

Therefore, the dilated line equation is given as:

`y=mx+b\\y=2x+8`