Answer:
Assuming that the angle is in radians, the velocity of the oscillator at
 would be equal to approximately
would be equal to approximately
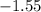 .
.
Step-by-step explanation:
Assume that the
 here stands for the displacement of the oscillator from its equilibrium position at time
here stands for the displacement of the oscillator from its equilibrium position at time
 .
.
Velocity is the first derivative of displacement with respect to time. So is the case in this question. Differentiate the expression for
 with respect to
with respect to
 to find the velocity at time
to find the velocity at time
 :
:
![v(t) = (d)/(dt) [x(t)] = (d)/(dt) [16.2\, \cos(4.68 \, t + 0.420)]= 16.2 \, (d)/(dt) [16.2\, \cos(4.68 \, t + 0.420)]](https://img.qammunity.org/2020/formulas/physics/middle-school/h7cc06q0z5to3n6arsoooua4mw3seq4167.png) .
.
In calculus,
![(d)/(dt) \,[\cos(f(t))] = - \sin(t) \cdot (d)/(dt)[f(t)]](https://img.qammunity.org/2020/formulas/physics/middle-school/eyndhuixojv557s7a8ma5pjm591fjjlpwi.png) by the chain rule. In this case the inner function is
by the chain rule. In this case the inner function is
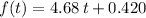 . Its first derivative is equal to
. Its first derivative is equal to
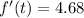 . Hence
. Hence
![(d)/(dt) [\cos(4.68 \, t + 0.420)] = - \sin(4.68\, t + 0.420) \cdot 4.68 = -4.68\, \sin(4.68\, t + 0.420)](https://img.qammunity.org/2020/formulas/physics/middle-school/bhi6rhsyyzpwjyq2cb71ksx40t05ovcim3.png) .
.
Therefore
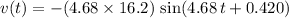 .
.
At time
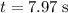 , that would be equal to
, that would be equal to
 .
.
Hence the (linear) velocity of the oscillator at
 would be equal to
would be equal to
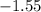 .
.