Question

Let a, b, c, and d be non-zero real numbers. If the quadratic equation ax(cx + d) = -b(cx+d) is solved for x, which of the following is a possible ratio of the 2 solutions?

(1) -ab/cd(2) -ac/bd(3) -ad/bc(4) ab/cd(5) ad/bc

Answer 1

Option d

Explanation:

given that a, b, c, and d be non-zero real numbers.

`ax(cx + d) = -b(cx+d) \\acx^2+x(ad+bc)+bd =0`

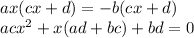
we can factorise this equation by grouping

`(acx^2+xad)+)xbc+bd) =0\\ax(cx+d) +b(cx+d) =0\\(ax+b)(cx+d) =0`

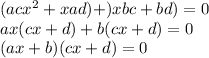
Equate each factor to 0 to get

`x=(-b)/(a) , (-d)/(c)`

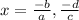
Ratio of one solution to another would be

`(-b)/(a) / (-d)/(c) \\=(ad)/(bc)`

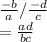
So ratio would be ad/bc

Out of the four options given, option d is equal to this

So option d is right