Answer:
a)30.14 rad/s2
b)43.5 rad/s
c)60633 J
d)42 kW
e)84 kW
Step-by-step explanation:
If we treat the propeller is a slender rod, then its moments of inertia is
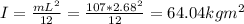
a. The angular acceleration is Torque divided by moments of inertia:
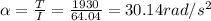
b. 5 revolution would be equals to
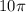 rad, or 31.4 rad. Since the engine just got started
rad, or 31.4 rad. Since the engine just got started
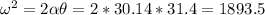
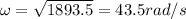
c. Work done during the first 5 revolution would be torque times angular displacement:
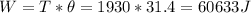
d. The time it takes to spin the first 5 revolutions is
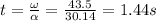
The average power output is work per unit time
 or 42 kW
or 42 kW
e.The instantaneous power at the instant of 5 rev would be Torque times angular speed at that time:
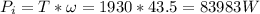 or 84 kW
or 84 kW