Answer:
(a) more than 2 such accidents in the next month
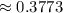
(b) more than 4 such accidents in the next 2 months
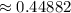
(c) more than 5 such accidents in the next 3 more than 5 such accidents in the next 3 months

Explanation:
Let N be the Random variable that marks the number of crashes in certain month.
Now let us use Poisson distribution since we are given with average number of crashes that is N \sim Pois(2.2)
(A) more than 2 such accidents in the next month
Probability(more than 2 such accidents in the next month)=P(N>2)
P(N>2)=1-P(N=0)-P(N=1)-P(N=2)
=>
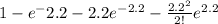
=>
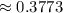
B) more than 4 such accidents in the next 2 months
since the average number of crashes in 1 month is 2.2, the average number of crashes in two months is 4.4. hence, if we say that
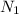 is the number of crashes in 2 months, we have that
is the number of crashes in 2 months, we have that
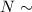 Pois(4.4)
Pois(4.4)
Thus,
Probability(more than 4 such accidents in the next 2 months)=P(
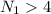 )
)
=
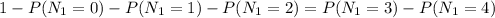
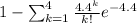
=>
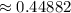
C) more than 5 such accidents in the next 3 more than 5 such accidents in the next 3 months
If we say that
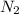 marks the number of crashes in the next 3 months , using the same argument as in (a) we have that a
marks the number of crashes in the next 3 months , using the same argument as in (a) we have that a
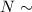 Pois(6.6)
Pois(6.6)
Hence
P(
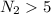 )=
)=

=>
