Answer:
a) The Energy added should be 484.438 MJ
b) The Kinetic Energy change is -484.438 MJ
c) The Potential Energy change is 968.907 MJ
Step-by-step explanation:
Let 'm' be the mass of the satellite , 'M'(6×
 be the mass of earth , 'R'(6400 Km) be the radius of the earth , 'h' be the altitude of the satellite and 'G' (6.67×
be the mass of earth , 'R'(6400 Km) be the radius of the earth , 'h' be the altitude of the satellite and 'G' (6.67×
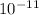 N/m) be the universal constant of gravitation.
N/m) be the universal constant of gravitation.
We know that the orbital velocity(v) for a satellite -
v=
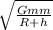 [(R+h) is the distance of the satellite from the center of the earth ]
[(R+h) is the distance of the satellite from the center of the earth ]
Total Energy(E) = Kinetic Energy(KE) + Potential Energy(PE)
For initial conditions ,
h =
 = 98 km = 98000 m
= 98 km = 98000 m
∴Initial Energy (
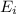 ) =
) =
 m
m
 +
+
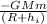
Substituting v=
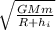 in the above equation and simplifying we get,
in the above equation and simplifying we get,
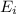 =
=
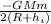
Similarly for final condition,
h=
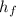 = 198km = 198000 m
= 198km = 198000 m
∴Final Energy(
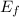 ) =
) =

a) The energy that should be added should be the difference in the energy of initial and final states -
∴ ΔE =
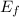 -
-
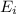
=
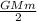 (
(
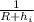 -
-
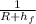 )
)
Substituting ,
M = 6 ×
 kg
kg
m = 1036 kg
G = 6.67 ×
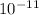
R = 6400000 m
 = 98000 m
= 98000 m
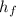 = 198000 m
= 198000 m
We get ,
ΔE = 484.438 MJ
b) Change in Kinetic Energy (ΔKE) =
 m[
m[
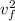 -
-
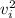 ]
]
=
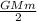 [
[
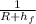 -
-
 ]
]
= -ΔE
= - 484.438 MJ
c) Change in Potential Energy (ΔPE) = GMm[
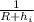 -
-
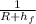 ]
]
= 2ΔE
= 968.907 MJ