Answer:
The base is decreasing at a rate of 7.8571 centimeters per minute.
Explanation:
We are given the following information in the question:
The height of a triangle is increasing at a rate of 2.5 cm/minute
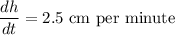
The area of the triangle is increasing at a rate of 2.5 square cm/minute.
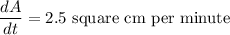
Area of triangle is given by:
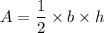
where A is the area of triangle, b is the base of triangle and h is the height of the triangle.
Differentiating, we get,
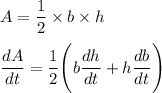
We have to find rate of change of base of the triangle when the altitude is 7 centimeters and the area is 84 square centimeters
h = 7 cm
A = 84 square centimeters
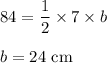
Putting the values, we get:
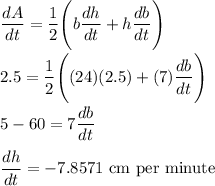
Thus, the base is decreasing at a rate of 7.8571 centimeters per minute.