Question

A roller coaster cart of mass m = 223 kg starts stationary at point A, where h1 = 26.8 m and a while later is at B, were h2 = 14.7 m. The acceleration of gravity is 9.8 m/s 2 . What is the speed of the cart at B, ignoring the effect of friction?

Answer 1

the guy above me is correct

Step-by-step explanation:

Answer 2

vB = 15.4 m/s

Step-by-step explanation:

Principle of conservation of energy:

Because there is no friction the mechanical energy is conserve

ΔE = 0

ΔE : mechanical energy change (J)

K : Kinetic energy (J)

U: Potential energy (J)

K = (1/2)mv²

U = m*g*h

Where :

m: mass (kg)

v : speed (m/s)

h : hight (m)

Ef - Ei = 0

(K+U)final - (K+U)initial =0

(K+U)final = (K+U)initial

((1/2)mv²+m*g*h)final = ((1/2)mv²+m*g*h)initial , We divided by m both sides of the equation:

((1/2)vB² + g*hB = (1/2 )vA²+ g*hA

(1/2) (vB)² + (9.8)*(14.7) = 0 + (9.8)(26.8 )

(1/2) (vB)² = (9.8)(26.8 ) - (9.8)*(14.7)

(vB)² = (2)(9.8)(26.8 - 14.7)

(vB)² = 237.16

`v_(B) = √(237.16)`

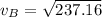
vB = 15.4 m/s : speed of the cart at B