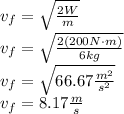Answer:
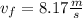
Step-by-step explanation:
First, we calculate the work done by this force after the box traveled 14 m, which is given by:
![W=\int\limits^(x_f)_(x_0) {F(x)} \, dx \\W=\int\limits^(14)_(0) ({18N-0.530(N)/(m)x}) \, dx\\W=[(18N)x-(0.530(N)/(m))(x^2)/(2)]^(14)_(0)\\W=(18N)14m-(0.530(N)/(m))((14m)^2)/(2)-(18N)0+(0.530(N)/(m))(0^2)/(2)\\W=252N\cdot m-52N\cdot m\\W=200N\cdot m](https://img.qammunity.org/2020/formulas/physics/high-school/x7jmag67v0o571957bdqxr32fcdr5wy0cx.png)
Since we have a frictionless surface, according to the the work–energy principle, the work done by all forces acting on a particle equals the change in the kinetic energy of the particle, that is:
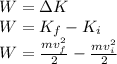
The box is initially at rest, so
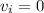 . Solving for
. Solving for
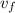 :
: