Question

Find the zeros of the polynomial function and state the multiplicity of each.

f(x) = 3(x + 8^)2(x - 8)^3


-8, multiplicity 2; 8, multiplicity 3

4, multiplicity 1; 8, multiplicity 1; -8, multiplicity 1

-8, multiplicity 3; 8, multiplicity 2

4, multiplicity 1; -8, multiplicity 3; 8, multiplicity 3

Answer 1

The zeros of the polynomial function f(x) = 3(x + 8)^2(x - 8)^3 are -8 with multiplicity 2, and 8 with multiplicity 3.

Step-by-step explanation:

The student is asking to find the zeros of the polynomial function f(x) = 3(x + 8)2(x - 8)3 and to state the multiplicity of each. To find the zeros of this function, we set f(x) equal to zero and solve for x:

• 3(x + 8)2(x - 8)3 = 0

We can see that there are two distinct zeros: -8 and 8. The multiplicity of each zero corresponds to the exponent on the factor in the polynomial:

• The zero -8 comes from the factor (x + 8)2, and so it has a multiplicity of 2.
• The zero 8 comes from the factor (x - 8)3, and so it has a multiplicity of 3.

Therefore, the zeros of the polynomial function f(x) are:

• -8, with multiplicity 2
• 8, with multiplicity 3

Answer 2

a) 8, multiplicity 2; 8, multiplicity 3

Step-by-step explanation:

Remember that a is a zero of the polynomial f(x) if f(a)=0 and has multiplicity n if the termn (x-a) is n times in the factorization of f(x).

We have that

`f(x)=3(x + 8)^2(x - 8)^3`

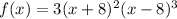
Observe that

1.
`f(-8)=3(-8 + 8)^2(-8 - 8)^3=3*0*(-16)^3=0`

and (x+8) appear two times in the factorization of f(x). Then -8 is a zero of f(x) with multiplicity 2.

2.
`f(8)=3(8 + 8)^2(8 - 8)^3=3*16^2*0^3=0`

and and (x - 8) appear three times in the factorization of f(x). Then 8 is a zero of f(x) with multiplicity 3.

Since f(x) has degree 5 and the sum of the multiplicities is 5 then f(x) hasn't more zeros.