Answer:
Option d
Step-by-step explanation:
From Maxwell's law, we know that;
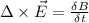
where
E = Electric Field
B = Magnetic Field
Also from Lenz Law:
emf, e = -
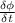
where
 = magnetic flux linkage
= magnetic flux linkage
Now, in order for the current not to be induced in the loop, option a and c do not hold.
Since from the above equations, variation in both or any of the magnetic and electric fields will result in the induction of current as both are vector.
For the option b, if we rotate the loop about the diameter or increase the size or area of the loop, it will result in the change in its magnetic flux and current will be induced as is clear from the given equation:
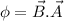
Now, in case of option d, if we consider a uniform magnetic field, then there won't be any variation and hence no current will be induced while we slide the wire perpendicular to the loop.