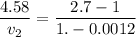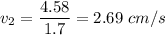Answer:2.69 cm/s
Step-by-step explanation:
We know that drag force
Fd=6 πμ r v
μ=Dynamic viscosity
r=radius
v=terminal velocity
Bouncy force
Fb= ρ V g

V=volume
At the condition of terminal velocity
when going down ( aluminum)
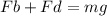
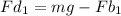 ---------1
---------1
when going up ( air)
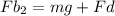
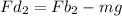 -----------2
-----------2
m =mass of object

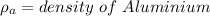
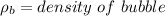
Divide 1 and 2
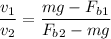
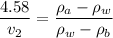
divide by
 in R.H.S
in R.H.S