Question

The average distance between collisions for atoms in a real gas is known as the mean free path (see pp. 298-9 in McKay). Which of the answers below best represents the mean free path for gas molecules at STP with atomic radii on the order of 1.0 x 10-10 m? The average distance between collisions for atoms in a real gas is known as the mean free path (see pp. 298-9 in McKay). Which of the answers below best represents the mean free path for gas molecules at STP with atomic radii on the order of 1.0 x 10-10 m?

A. 30 nm
B. 3000 nm
C. 300 nm
D. 3 nm

Answer 1

300 nm

Step-by-step explanation:

R = Gas constant = 8.314 J/molK

r = Atomic radii =
`1* 10^(-10)\ m`

d = Atomic diameter =
`2r=2* 10^(-10)\ m`

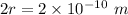
At STP

T = Temperature = 273.15 K

P = Pressure = 100 kPa

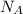`N_A` = Avogadro's number =
`6.022* 10^(23)`

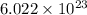
The mean free path is given by

`\lambda=(RT)/(\sqrt2d^2N_AP)\\\Rightarrow \lambda=(8.314* 273.15)/(\sqrt2 \pi * (2* 10^(-10))^2* 6.022* 10^(23)* 100000)\\\Rightarrow \lambda=2.12165* 10^(-7)\ m=212.165* 10^(-9)\ m=212.165\ nm`

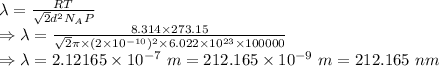
The answer that best represents the mean free path for gas molecules is 300 nm