Answer:
The sample size should be 122,150 and the sample size is not practical.
Explanation:
Consider the provided information.
The sample mean is within 0.01 of the population mean.
E=0.01
σ=1.5
Confidence level is 98%
1-α=0.98
α=1-0.98=0.02
Formula for sample size is:
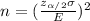
By using the normal probability table:

Substitute the respective values in the above formula.

Sample size doesn't seem practical as 122,150 is extremely large number.