Question

A manufacturer knows that their items have a normally distributed length, with a mean of 7.1 inches, and standard deviation of 1.7 inches.Round your answer to four decimals.If 24 items is chosen at random, what is the probability that their mean length is less than 6.2 inches?

Answer 1

0.0047

step-by-step explanation

Answer 2

Answer: 0.0047

Explanation:

Given : A manufacturer knows that their items have a normally distributed length, with a mean of 7.1 inches, and standard deviation of 1.7 inches.

i.e.
`\mu=7.1\text{ inches}`

`\sigma=17\text{ inches}`

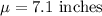
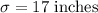
Sample size : n= 24

Let
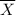`\overline{X}` be the sample mean.

Formula :
`z=\frac{\overline{x}-\mu}{(\sigma)/(√(n))}`

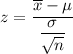
Then, the probability that their mean length is less than 6.2 inches will be :-

`P(\overline{x}<6.2)=P(\frac{\overline{x}-\mu}{(\sigma)/(√(n))}<(6.2-7.1)/((1.7)/(√(24))))\\\\\approx P(z<-2.6)\\\\=1-P(z<2.6)\ \ [\because\ P(Z<-z)=1-P(Z<z)]\\\\=1-0.9953=0.0047\ \ \ [ \text{Using z-value table}]`

hence,. the required probability = 0.0047