Question

A landscape architect wished to enclose a rectangular garden on one side by a brick wall costing $20/ft and on the other three sides by a metal fence costing $10/ft. If the area of the garden is 122 square feet, find the dimensions of the garden that minimize the cost.

Answer 1

The dimensions of the garden that minimize the cost is 9.018 feet(length) and 13.528 feet(width)

Explanation:

Let the length of garden be x

Let the breadth of garden be y

Area of Rectangular garden =
`Length * Breadth = xy`

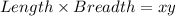
We are given that the area of the garden is 122 square feet

So,
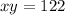`xy=122` ---A

A landscape architect wished to enclose a rectangular garden on one side by a brick wall costing $20/ft

So, cost of brick along length x = 20 x

On the other three sides by a metal fence costing $10/ft.

So, Other three side s = x+2y

So, cost of brick along the other three sides= 10(x+2y)

So, Total cost = 20x+10(x+2y)=20x+10x+20y=30x+20y

Total cost = 30x+20y

Substitute the value of y from A

Total cost =
`30x+20((122)/(x))`

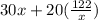
Total cost =
`(2440)/(x)+30x`

Now take the derivative to minimize the cost

`f(x)=(2440)/(x)+30x`

`f'(x)=-(2440)/(x^2)+30`

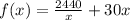
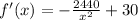
Equate it equal to 0

`0=-(2440)/(x^2)+30`

`(2440)/(x^2)=30`

`\sqrt{(2440)/(30)}=x`

`9.018 =x`

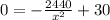
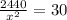
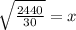
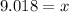
Now check whether it is minimum or not

take second derivative

`f'(x)=-(2440)/(x^2)+30`

`f''(x)=-(-2)(2440)/(x^3)`

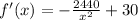
Substitute the value of x

`f''(x)=-(-2)(2440)/((9.018)^3)`

`f''(x)=6.6540`

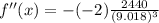
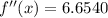
Since it is positive ,So the x is minimum

Now find y

Substitute the value of x in A

`(9.018)y=122`

`y=(122)/(9.018)`

`y=13.528`

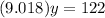
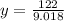
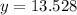
Hence the dimensions of the garden that minimize the cost is 9.018 feet(length) and 13.528 feet(width)