Answer:
The given function is an odd function.
Explanation:
We define a function f(x) as even function when f(-x) = f(x) and odd function when f(-x) = - f(x) and otherwise it is neither even nor odd function.
Now, we are given a function of x as
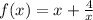 and we have to deternime whether the function f(x) is even, odd, or neither even nor odd.
and we have to deternime whether the function f(x) is even, odd, or neither even nor odd.
Now,
![f(-x) = - x + (4)/(- x) = - x - (4)/(x) = - [x + (4)/(x)] = - f(x)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/a1wzlflrgfdiufj2i0vbv20t16mtyfiscu.png)
Therefore, the given function is an odd function. (Answer)